| Symmetry in Physics |
Above: the tracks of pairs of electrons and their antimatter equivalent, positrons, being produced from high-
energy photons (such as gamma rays) like those that may be obtained in a bubble chamber. A bubble chamber
is a particle tracker that only detects electrically charged particles, and so the photons leave no visible tracks. In
the upper diagram, the track of the photon is shown by the black dotted line. Electron tracks are shown in blue,
positron tracks in red (in reality the tracks are of course colourless!). In the lower diagram, the main trace shows
an electron-positron pair being produced. The positron then annihilates with an atomic electron (in the bubble
chamber’s liquid), producing another high-energy photon, whose track is invisible but can be estimated (black
dotted line) which produces another electron-positron pair. See an example here: http://www.sciencephoto.
com/media/1385/enlarge# (courtesy of an x-tutor of mine).
Q. If the tracks shown in red represent the positrons, and the magnetic fields are uniform and pointing into or
out of the screen, then what is the direction of the magnetic field, into or out of the screen, in each case?
According to the Lorentz force law, the force acting on a particle moving through a uniform magnetic field is
given by:
energy photons (such as gamma rays) like those that may be obtained in a bubble chamber. A bubble chamber
is a particle tracker that only detects electrically charged particles, and so the photons leave no visible tracks. In
the upper diagram, the track of the photon is shown by the black dotted line. Electron tracks are shown in blue,
positron tracks in red (in reality the tracks are of course colourless!). In the lower diagram, the main trace shows
an electron-positron pair being produced. The positron then annihilates with an atomic electron (in the bubble
chamber’s liquid), producing another high-energy photon, whose track is invisible but can be estimated (black
dotted line) which produces another electron-positron pair. See an example here: http://www.sciencephoto.
com/media/1385/enlarge# (courtesy of an x-tutor of mine).
Q. If the tracks shown in red represent the positrons, and the magnetic fields are uniform and pointing into or
out of the screen, then what is the direction of the magnetic field, into or out of the screen, in each case?
According to the Lorentz force law, the force acting on a particle moving through a uniform magnetic field is
given by:

A uniform magnetic field is one which has the same numeric value and direction at all points in space (in the
region of interest). The force, velocity and magnetic fields are all vectors – they have direction as well as
numerical values. The x refers to the vector cross-product of v and B. This cross-product takes the component of
the particle’s velocity that is at right-angles to the magnetic field lines and uses this to calculate the force on the
particle (there is no force on a particle moving parallel or antiparallel to a uniform magnetic field).
The direction of the force can be obtained using the right-hand rule. Take your right-hand and point the thumb
straight upwards, this will represent the direction of the force. Point your index finger straight forwards, this
represents the direction of the velocity (strictly that component of it perpendicular to the magnetic field) and then
point your middle finger straight to your left, this represents the direction of the magnetic field.
Lets look at the lower diagram first, the (invisible) photons are moving straight upwards from the bottom of the
picture, along the positive y-axis. Now, orient your right-hand so that your middle finger points into the screen and
your index finger points along the initial trajectory of the particle (straight up, along the positive y-axis). Your
thumb, which should now be pointing left (along the negative x-axis) shows the direction of the force acting on a
positive charge, i.e. acting on the positron.
The red tracks curve to the left (anticlockwise) and so are the positrons. negative electric charges are pushed in
the direction opposite your thumb and spiral to the right (clockwise).
Q. If the red tracks again represent positrons, then is the magnetic field in the top diagram pointing into or out of
the screen?
Notice that the spirals get tighter – their radius diminishes and then the track ends. This is because the
particles are losing energy – they radiate away photons when they move in circles and slow down. Faster moving
particles travel further in a given time interval and so curve less over a given distance, but as they slow down, the
curves they trace out have smaller radii.
Why Symmetry? A brief overview of the history of philosophy:
Symmetry is crucial in physics. The ancients noticed the importance of symmetry in their philosophy or
metaphysics. They distinguished four directions, N, E, S and W and an opposing pair of up and down. From at
least the time of the ancient Greeks, the most learned sages of the ancient world taught that matter was
comprised of four elements: fire, earth, air and water. These elements could be grouped into opposing pairs: fire
(hot and dry) and water (cool and moist); air (warm and moist) and earth (cold and dry). The four elements could
also be united into the mysterious 5th element, the element of spirit, the hidden light in all things from which all
things, including the four elements were made and into which they could be recombined. Alchemy (which was not
really about making gold!) drew heavily upon this ancient philosophy, including such sources as the hermetic
Corpus and the Gospels.
Alchemical emblems played on symmetry in very artistic ways, including the use of trees to combine the world
above with the world below; an idea that manifested in medieval times in the Qabalistic Tree of Life, so popular in
modern culture. This philosophy taught how creation came about through a breaking of symmetry, from a perfect
unity into a duality and then into four opposing forces, and so on, spanning 10 or 11 dimensions.
Of course, intuition can only go so far, and the ancients did not get everything right. However, to be fair to them
their model was not wrong, no more than Newtonian mechanics is wrong in failing to predict relativistic effects at
very high speeds. It was a model, and like all models it had its merits and its limitations.
Another symmetry was seen between inner and outer realities. In particular, the ancients at first did not clearly
distinguish between the objective external world and the inner psychological world, indeed they often held that
the former was illusory and only the latter was real, in contrast to modern science which arguably adopts the
opposite view. However, alchemists held very different views – some were proto-scientists, focusing on the
physical make-up of matter, sometimes in the hope of turning lead into gold! Others considered this impossible
and were instead more concerned with psychology and mystical philosophy and to these alchemists the elements
and chemicals paralleled the spiritual world in many ways. They even devised various ideas about how the
Universe was created.
Despite the limitations of their time, the alchemists at least strove to understand the world and to apply ever more
rigorous science to it; a science based at least in part upon empirical observations, and some were highly skilled
chemists. It is remarkable how intuitively they came to conclusions that parallel in many ways the discovery of
modern physics, with its four fundamental forces, virtual photons (hidden light) binding atoms together and the
numerous dimensions of string theory. Perhaps it is not so surprising, since both alchemists and scientists have
been motivated by the same basic principle – symmetry.
What is the most symmetrical 3D shape? Most people would probably think of complicated fractals, but in fact one
can get no more symmetrical than a sphere. A sphere has an infinite number of planes of symmetry and an
infinite number of axes of rotational symmetry. In a similar fashion, empty space can be thought of as the most
symmetric state – a state of nothing! The complex world we see came about not by increasing symmetry, but by
breaking it. This idea has lead to the modern scientific hypothesis of Grand Unification – the idea that at
sufficiently high energies (akin to the first fraction of a second after the Big Bang) the fundamental forces of
Nature were indistinguishable and matter comprised elementary particles that were all indistinguishable from one-
another; a state of unity and perfect or near-perfect symmetry.
Background - Quantum Mechanics (QM)
In quantum mechanics we describe the behaviour of a system by a wave equation. If we are dealing with non-
relativistic energies and velocities (i.e. speeds much less than the speed of light) and ignoring particle spin, we
can use Schrodinger’s wave equation (either the time-dependent form or the time-independent form for
stationary states):
region of interest). The force, velocity and magnetic fields are all vectors – they have direction as well as
numerical values. The x refers to the vector cross-product of v and B. This cross-product takes the component of
the particle’s velocity that is at right-angles to the magnetic field lines and uses this to calculate the force on the
particle (there is no force on a particle moving parallel or antiparallel to a uniform magnetic field).
The direction of the force can be obtained using the right-hand rule. Take your right-hand and point the thumb
straight upwards, this will represent the direction of the force. Point your index finger straight forwards, this
represents the direction of the velocity (strictly that component of it perpendicular to the magnetic field) and then
point your middle finger straight to your left, this represents the direction of the magnetic field.
Lets look at the lower diagram first, the (invisible) photons are moving straight upwards from the bottom of the
picture, along the positive y-axis. Now, orient your right-hand so that your middle finger points into the screen and
your index finger points along the initial trajectory of the particle (straight up, along the positive y-axis). Your
thumb, which should now be pointing left (along the negative x-axis) shows the direction of the force acting on a
positive charge, i.e. acting on the positron.
The red tracks curve to the left (anticlockwise) and so are the positrons. negative electric charges are pushed in
the direction opposite your thumb and spiral to the right (clockwise).
Q. If the red tracks again represent positrons, then is the magnetic field in the top diagram pointing into or out of
the screen?
Notice that the spirals get tighter – their radius diminishes and then the track ends. This is because the
particles are losing energy – they radiate away photons when they move in circles and slow down. Faster moving
particles travel further in a given time interval and so curve less over a given distance, but as they slow down, the
curves they trace out have smaller radii.
Why Symmetry? A brief overview of the history of philosophy:
Symmetry is crucial in physics. The ancients noticed the importance of symmetry in their philosophy or
metaphysics. They distinguished four directions, N, E, S and W and an opposing pair of up and down. From at
least the time of the ancient Greeks, the most learned sages of the ancient world taught that matter was
comprised of four elements: fire, earth, air and water. These elements could be grouped into opposing pairs: fire
(hot and dry) and water (cool and moist); air (warm and moist) and earth (cold and dry). The four elements could
also be united into the mysterious 5th element, the element of spirit, the hidden light in all things from which all
things, including the four elements were made and into which they could be recombined. Alchemy (which was not
really about making gold!) drew heavily upon this ancient philosophy, including such sources as the hermetic
Corpus and the Gospels.
Alchemical emblems played on symmetry in very artistic ways, including the use of trees to combine the world
above with the world below; an idea that manifested in medieval times in the Qabalistic Tree of Life, so popular in
modern culture. This philosophy taught how creation came about through a breaking of symmetry, from a perfect
unity into a duality and then into four opposing forces, and so on, spanning 10 or 11 dimensions.
Of course, intuition can only go so far, and the ancients did not get everything right. However, to be fair to them
their model was not wrong, no more than Newtonian mechanics is wrong in failing to predict relativistic effects at
very high speeds. It was a model, and like all models it had its merits and its limitations.
Another symmetry was seen between inner and outer realities. In particular, the ancients at first did not clearly
distinguish between the objective external world and the inner psychological world, indeed they often held that
the former was illusory and only the latter was real, in contrast to modern science which arguably adopts the
opposite view. However, alchemists held very different views – some were proto-scientists, focusing on the
physical make-up of matter, sometimes in the hope of turning lead into gold! Others considered this impossible
and were instead more concerned with psychology and mystical philosophy and to these alchemists the elements
and chemicals paralleled the spiritual world in many ways. They even devised various ideas about how the
Universe was created.
Despite the limitations of their time, the alchemists at least strove to understand the world and to apply ever more
rigorous science to it; a science based at least in part upon empirical observations, and some were highly skilled
chemists. It is remarkable how intuitively they came to conclusions that parallel in many ways the discovery of
modern physics, with its four fundamental forces, virtual photons (hidden light) binding atoms together and the
numerous dimensions of string theory. Perhaps it is not so surprising, since both alchemists and scientists have
been motivated by the same basic principle – symmetry.
What is the most symmetrical 3D shape? Most people would probably think of complicated fractals, but in fact one
can get no more symmetrical than a sphere. A sphere has an infinite number of planes of symmetry and an
infinite number of axes of rotational symmetry. In a similar fashion, empty space can be thought of as the most
symmetric state – a state of nothing! The complex world we see came about not by increasing symmetry, but by
breaking it. This idea has lead to the modern scientific hypothesis of Grand Unification – the idea that at
sufficiently high energies (akin to the first fraction of a second after the Big Bang) the fundamental forces of
Nature were indistinguishable and matter comprised elementary particles that were all indistinguishable from one-
another; a state of unity and perfect or near-perfect symmetry.
Background - Quantum Mechanics (QM)
In quantum mechanics we describe the behaviour of a system by a wave equation. If we are dealing with non-
relativistic energies and velocities (i.e. speeds much less than the speed of light) and ignoring particle spin, we
can use Schrodinger’s wave equation (either the time-dependent form or the time-independent form for
stationary states):

The time-independent form is used when there is no change in a system over time, such as the electron in a
hydrogen atom when it is in one of the stationary states following a measurement, such as a 1s orbital. In a
stationary state there is no variation in observable properties over time – the electron will still be in the 1s state
should it be measured again. (That is not to say that there is never any further change in time – when the atom
is perturbed by some further interaction with some particle or suitable measurement, then its state may change).
The wave function describes the properties of the system in its current state and any possible values of
observable properties that the system may have. The wave function contains all the information we can know
about the system. We use mathematical operators to extract the information of interest from the system. For
example, the Hamiltonian operator extracts information about the energy of the system following a measurement
of energy (how else could we find out its energy?). The measurement actually changes the system.
The ideal wave function contains all the information we can know about the system. The solutions to Schrődinger’
s equation are wave functions (eigenfunctions or linear combinations of eigenfunctions). This can be explained
by wave-particle duality – all particles behave like waves (or groups of waves) which will give rise to particle-like
behaviour under certain conditions. Schrodinger’s equation ignores spin and does not give reliable wave
functions for relativistic energies. For relativistic conditions we have to use either the Klein-Gordon equation (for
particles with zero spin) or Dirac’s equation (for particles with spin) which are more complex.
Schrodinger’s wave equation is sometimes written in terms of the Hamiltonian operator:
hydrogen atom when it is in one of the stationary states following a measurement, such as a 1s orbital. In a
stationary state there is no variation in observable properties over time – the electron will still be in the 1s state
should it be measured again. (That is not to say that there is never any further change in time – when the atom
is perturbed by some further interaction with some particle or suitable measurement, then its state may change).
The wave function describes the properties of the system in its current state and any possible values of
observable properties that the system may have. The wave function contains all the information we can know
about the system. We use mathematical operators to extract the information of interest from the system. For
example, the Hamiltonian operator extracts information about the energy of the system following a measurement
of energy (how else could we find out its energy?). The measurement actually changes the system.
The ideal wave function contains all the information we can know about the system. The solutions to Schrődinger’
s equation are wave functions (eigenfunctions or linear combinations of eigenfunctions). This can be explained
by wave-particle duality – all particles behave like waves (or groups of waves) which will give rise to particle-like
behaviour under certain conditions. Schrodinger’s equation ignores spin and does not give reliable wave
functions for relativistic energies. For relativistic conditions we have to use either the Klein-Gordon equation (for
particles with zero spin) or Dirac’s equation (for particles with spin) which are more complex.
Schrodinger’s wave equation is sometimes written in terms of the Hamiltonian operator:

The Hamiltonian in classical physics is the total energy of the system being considered. In quantum mechanics
(QM) the hamiltonian is replaced by the Hamiltonian operator. A QM operator is a mathematical operation
that extracts required information from the wave function. {Operators in mathematics simply perform predefined
operations, such as the addition operator, +, or the differential operator, d/dx). This information must correspond
to an observable property, such as energy in the case of the Hamiltonian operator. The Hamiltonian operator
extracts the energy, E, that the system may possess for each given eigenfunction. This energy is called the
eigenvalue of the eigenstate. The eigenfunction is also called an eigenstate as it describes the system in one
of its possible (stationary) states. For many systems there is more than wave function solution to the wave
equation. For example, in a hydrogen atom, the electron can exist in one of a number of possible eigenstates (or
a mixture of eigenstates) such as the 1s orbital or the 2p orbital, for example. The Hamiltonian operator then
gives us the energy of the corresponding state, such as the measurable energy of the electron when in the 2p
state. These predictions can of course be verified by experiment and the predictions made by models of the
hydrogen atom are astonishingly accurate. This is important, since Schrodinger’s equation can not be
mathematically derived – it was an intuitive estimate that turned out to be correct 9except for spin and
corrections for relativistic energies) however, it works!
There is quite a number of operators in QM, and they can be represented as matrices. To give observable
eigenvalues they must be matrices that have a set of real eigenvalues (not complex or imaginary eigenvalues)
and such matrices are called Hermitian matrices and our operators are Hermitian operators.
Simultaneous Eigenvalues
Consider two arbitrary operators, call them operate P and operator Q, with eigenvalues p and q respectively:
(QM) the hamiltonian is replaced by the Hamiltonian operator. A QM operator is a mathematical operation
that extracts required information from the wave function. {Operators in mathematics simply perform predefined
operations, such as the addition operator, +, or the differential operator, d/dx). This information must correspond
to an observable property, such as energy in the case of the Hamiltonian operator. The Hamiltonian operator
extracts the energy, E, that the system may possess for each given eigenfunction. This energy is called the
eigenvalue of the eigenstate. The eigenfunction is also called an eigenstate as it describes the system in one
of its possible (stationary) states. For many systems there is more than wave function solution to the wave
equation. For example, in a hydrogen atom, the electron can exist in one of a number of possible eigenstates (or
a mixture of eigenstates) such as the 1s orbital or the 2p orbital, for example. The Hamiltonian operator then
gives us the energy of the corresponding state, such as the measurable energy of the electron when in the 2p
state. These predictions can of course be verified by experiment and the predictions made by models of the
hydrogen atom are astonishingly accurate. This is important, since Schrodinger’s equation can not be
mathematically derived – it was an intuitive estimate that turned out to be correct 9except for spin and
corrections for relativistic energies) however, it works!
There is quite a number of operators in QM, and they can be represented as matrices. To give observable
eigenvalues they must be matrices that have a set of real eigenvalues (not complex or imaginary eigenvalues)
and such matrices are called Hermitian matrices and our operators are Hermitian operators.
Simultaneous Eigenvalues
Consider two arbitrary operators, call them operate P and operator Q, with eigenvalues p and q respectively:

These operators commute if when both are applied in turn, the order in which they are applied does not matter.
Operators are usually written with a ‘pointed hat’ above them.
If the two operators do commute in this way, then we write this as the commutator is 0:
Operators are usually written with a ‘pointed hat’ above them.
If the two operators do commute in this way, then we write this as the commutator is 0:

and there exists a set of eigenvalues of one operator, say set {p} that is also a set of eigenvalues of operator q,
a set of simultaneous eigenvalues for both operators. (Not all the eigenvalues of P are also eigenvalues of Q,
but a set of common eigenvalues can be found.) These common eigenvalues, pq (= qp) tell us that a state can
have unique values of both p and q.
As an example, the linear momentum (p) and energy (Hamiltonian, H) operators of a free particle do commute. A
free particle is a particle that is not interacting with a potential (a potential force field, such as an electric force
field) and I specified a free particle simply because the Hamiltonian operator is different depending upon the
potential and must be derived for each system being considered. (The Hamiltonian operator consists of a kinetic
energy operator and a potential energy operator). We will not go through the maths, but if we did then we need
to specify the potential and since for a free particle this is equal to zero then this is the simplest case. In this case
the operators do commute, that is:
a set of simultaneous eigenvalues for both operators. (Not all the eigenvalues of P are also eigenvalues of Q,
but a set of common eigenvalues can be found.) These common eigenvalues, pq (= qp) tell us that a state can
have unique values of both p and q.
As an example, the linear momentum (p) and energy (Hamiltonian, H) operators of a free particle do commute. A
free particle is a particle that is not interacting with a potential (a potential force field, such as an electric force
field) and I specified a free particle simply because the Hamiltonian operator is different depending upon the
potential and must be derived for each system being considered. (The Hamiltonian operator consists of a kinetic
energy operator and a potential energy operator). We will not go through the maths, but if we did then we need
to specify the potential and since for a free particle this is equal to zero then this is the simplest case. In this case
the operators do commute, that is:

and there is a set of simultaneous eigenvalues that can assign unique values to both the momentum and energy
of our particle for a certain set of eigenstates (wave functions).
If the particle is bound in a potential well (that is confined to have certain energy values and positions by an
energy potential, such as an electron bound to a proton by the Coulomb attraction in a hydrogen atom) then the
linear momentum and Hamiltonian operators no longer commute. The kinetic energy operator part of the
Hamiltonian operator does commute with the linear momentum, as it did with the free particle, but the potential
energy operator does not. This means that we can not find a set of states in which the particle has well-defined
momentum and well-defined energy.
Uncertainty Relations
Typically, when two operators do not commute, their observables exhibit an uncertainty relation. An example of
this is linear momentum and position (displacement) along the same axis. These two operators do not commute
along the x-axis:
of our particle for a certain set of eigenstates (wave functions).
If the particle is bound in a potential well (that is confined to have certain energy values and positions by an
energy potential, such as an electron bound to a proton by the Coulomb attraction in a hydrogen atom) then the
linear momentum and Hamiltonian operators no longer commute. The kinetic energy operator part of the
Hamiltonian operator does commute with the linear momentum, as it did with the free particle, but the potential
energy operator does not. This means that we can not find a set of states in which the particle has well-defined
momentum and well-defined energy.
Uncertainty Relations
Typically, when two operators do not commute, their observables exhibit an uncertainty relation. An example of
this is linear momentum and position (displacement) along the same axis. These two operators do not commute
along the x-axis:

This means that both the position and momentum of a particle can not be exactly determined – a system can not
have exact values of both momentum and position, in principle. (This has nothing to do with our inability to
conduct perfectly accurate measurements, but is instead a fundamental property of the system). This gives rise
to Heisenberg’s uncertainty relation:
have exact values of both momentum and position, in principle. (This has nothing to do with our inability to
conduct perfectly accurate measurements, but is instead a fundamental property of the system). This gives rise
to Heisenberg’s uncertainty relation:

Which tells us that the uncertainty in position, Delta x, multiplied by the uncertainty in momentum, Delta p, can
not be smaller than about ħ = h/2p, where h is Planck’s constant (h is approximately 6.626 x 10^-34 Joule
seconds).
Translational Invariance and Momentum Conservation
In geometry to translate a system or object means to move it along a straight line from one position in space to
another. For example we could move a spaceship along a straight line in any direction in 3D space. Not
surprisingly, we would expect the laws of physics on our spaceship to be the same at its new coordinates as at
its original coordinates. The laws of physics exhibit translational invariance – they do not depend on position in
space. To the very high degree currently measurable, this translational invariance is found to be quite correct.
There is the possibility that the laws of physics vary from region to region in the Universe, but certainly in the
visible part of the Universe there is no variation that has yet been noticed.
Just as the Hamiltonian operator extracts the energy of a state from a possible wave function describing that
state, so there are operators that can extract the linear momentum, the angular momentum, and other
properties, including positional information about the system after a translation in space.
When looking at the effects of translating a system we use an operator, D, which translates the coordinates of
the system, changing it from its original state to a new state obtained by measurement. If the Hamiltonian is not
changed by the translation (which it could be if the particle is in a directional force field) then we find that the
translation operator and the Hamiltonian commute:
not be smaller than about ħ = h/2p, where h is Planck’s constant (h is approximately 6.626 x 10^-34 Joule
seconds).
Translational Invariance and Momentum Conservation
In geometry to translate a system or object means to move it along a straight line from one position in space to
another. For example we could move a spaceship along a straight line in any direction in 3D space. Not
surprisingly, we would expect the laws of physics on our spaceship to be the same at its new coordinates as at
its original coordinates. The laws of physics exhibit translational invariance – they do not depend on position in
space. To the very high degree currently measurable, this translational invariance is found to be quite correct.
There is the possibility that the laws of physics vary from region to region in the Universe, but certainly in the
visible part of the Universe there is no variation that has yet been noticed.
Just as the Hamiltonian operator extracts the energy of a state from a possible wave function describing that
state, so there are operators that can extract the linear momentum, the angular momentum, and other
properties, including positional information about the system after a translation in space.
When looking at the effects of translating a system we use an operator, D, which translates the coordinates of
the system, changing it from its original state to a new state obtained by measurement. If the Hamiltonian is not
changed by the translation (which it could be if the particle is in a directional force field) then we find that the
translation operator and the Hamiltonian commute:

Mathematically this reduces to the following commutation between the linear momentum operator, p, and the
Hamiltonian:
Hamiltonian:

This is telling us that linear momentum is conserved. This is because the eigenvalues of the Hamiltonian
operator describe stationary states in which the energy does not vary with time and so, therefore, the linear
momentum is also not varying with time – the total linear momentum of a closed system is indeed conserved.
Rotational Invariance and Angular Momentum
In QM a particle can have two types of angular momentum – that due to its motion in a potential, such as an
electron ‘orbiting’ the nucleus of an atom, the so-called orbital angular momentum, L, and that due to its own
intrinsic ‘rotation’ about its axis, called spin (symbol s for a particle, S for the total spin of a system of several
particles). The total angular momentum, J is then given by:
J = L + S
In reality, the classical analogies of orbital and rotational angular momentum are not entirely correct, since in
QM particles do not follow definite trajectories (think of Heisenberg’s uncertainty principle – momentum and
position can not be both exact values). However, particles do have their QM equivalent of angular momentum,
which is quantised (can only take certain well-defined values). [In the ‘classical limit’ a large system of many
particles, such as a football, can have so many different values of angular momentum, that its range of
valuables is essentially continuous, as we experience it to be.]
Similar to linear momentum, total angular momentum is also conserved and when the Hamiltonian is not
changed by rotation:
operator describe stationary states in which the energy does not vary with time and so, therefore, the linear
momentum is also not varying with time – the total linear momentum of a closed system is indeed conserved.
Rotational Invariance and Angular Momentum
In QM a particle can have two types of angular momentum – that due to its motion in a potential, such as an
electron ‘orbiting’ the nucleus of an atom, the so-called orbital angular momentum, L, and that due to its own
intrinsic ‘rotation’ about its axis, called spin (symbol s for a particle, S for the total spin of a system of several
particles). The total angular momentum, J is then given by:
J = L + S
In reality, the classical analogies of orbital and rotational angular momentum are not entirely correct, since in
QM particles do not follow definite trajectories (think of Heisenberg’s uncertainty principle – momentum and
position can not be both exact values). However, particles do have their QM equivalent of angular momentum,
which is quantised (can only take certain well-defined values). [In the ‘classical limit’ a large system of many
particles, such as a football, can have so many different values of angular momentum, that its range of
valuables is essentially continuous, as we experience it to be.]
Similar to linear momentum, total angular momentum is also conserved and when the Hamiltonian is not
changed by rotation:

Linear momentum conservation was shown to be due to the translational invariance of physical systems.
Angular momentum is similarly due to rotational invariance. This means that the physics of a system do not
change if the spatial coordinates of the system are rotated in space – our spaceship behaves the same in
space, no matter what its orientation is (it would be quite mysterious otherwise!).
Parity
The parity transformation is another coordinate transformation and involves reflection (inversion) through the
origin of all the spatial positions of a system’s parts. This means that all x-values, for example, change to –x, so
that the spatial coordinate axes are inverted. This is not to say that we would necessarily actually carry out
such a transformation on a system, but we could configure two machines in which each is the mirror-image of
the other, or more specifically the inversion through the centre of the other system. It generally wouldn’t matter
whether or not all the cogs turned clockwise or anticlockwise, the system will be governed by the same physical
laws. (Of course its behaviour may be crucially different – a clock whose hands run in reverse is not very
useful to us, but its physics is the same as a normal clock!).
For the strong force and the electromagnetic force, it turns out that if the Hamiltonian is unchanged by the
parity transformation then:
Angular momentum is similarly due to rotational invariance. This means that the physics of a system do not
change if the spatial coordinates of the system are rotated in space – our spaceship behaves the same in
space, no matter what its orientation is (it would be quite mysterious otherwise!).
Parity
The parity transformation is another coordinate transformation and involves reflection (inversion) through the
origin of all the spatial positions of a system’s parts. This means that all x-values, for example, change to –x, so
that the spatial coordinate axes are inverted. This is not to say that we would necessarily actually carry out
such a transformation on a system, but we could configure two machines in which each is the mirror-image of
the other, or more specifically the inversion through the centre of the other system. It generally wouldn’t matter
whether or not all the cogs turned clockwise or anticlockwise, the system will be governed by the same physical
laws. (Of course its behaviour may be crucially different – a clock whose hands run in reverse is not very
useful to us, but its physics is the same as a normal clock!).
For the strong force and the electromagnetic force, it turns out that if the Hamiltonian is unchanged by the
parity transformation then:

So that parity is conserved. It turns out, however, that for the weak force, parity is not conserved. Thus, parity
conservation is only approximate. The reason for this comes back to our clock. Think of a corkscrew – it only
works when rotated in one sense (either clockwise or anticlockwise depending on the handedness of the
screw). [A property exploited by bacterial flagella.] A spinning particle, like an electron, that is also moving as a
whole follows a helical ‘path’ (not a strict trajectory but the QM equivalent) and the handedness of this path,
which depends on the direction of spin of the electron, affects weak force interactions. Thus, a parity
transformation can change the behaviour of weak force interactions which are said to violate parity
conservation. This property of the electron, or other particle with spin, is called helicity.
It happens that mathematical functions can be affected by a parity change in their coordinates in one of two
ways. Even functions are not changed at all, for example, the cosine of 180 degrees equals the cosine of -180
degrees equals -1. Odd functions change sign, for example the sine of 90 degrees equals 1, but the sine of
-90 degrees equals -1. The same is true of the wave functions describing particles. If the sign is unchanged by
a parity transform, then the particle is said to have even parity, or a parity of +1, for example the parity of the
proton is +1. If the wave function changes sign then the particle has odd parity (a parity of -1), for example, the
photon and the pion (pi meson). [There are thus two eigenvalues of the parity operator: +1 and -1.] Parity is
thus an intrinsic property of elementary particles. Anti-particles have opposite parity (opposite in sign) to their
corresponding particles. Applying parity transformations twice in succession returns the original system, i.e.
leaves the system unchanged.
Charge Conjugation
Charge conjugation (C) is a type of theoretic transformation in which all particles are replaced by their anti-
particles (and anti-particles by their particles) whilst leaving other properties unchanged. This changes the
sign of the particle’s electric charge, parity and magnetic moment, but leaves properties like momentum and
position unchanged.
Again for the strong and electromagnetic forces, charge conjugation is conserved:
conservation is only approximate. The reason for this comes back to our clock. Think of a corkscrew – it only
works when rotated in one sense (either clockwise or anticlockwise depending on the handedness of the
screw). [A property exploited by bacterial flagella.] A spinning particle, like an electron, that is also moving as a
whole follows a helical ‘path’ (not a strict trajectory but the QM equivalent) and the handedness of this path,
which depends on the direction of spin of the electron, affects weak force interactions. Thus, a parity
transformation can change the behaviour of weak force interactions which are said to violate parity
conservation. This property of the electron, or other particle with spin, is called helicity.
It happens that mathematical functions can be affected by a parity change in their coordinates in one of two
ways. Even functions are not changed at all, for example, the cosine of 180 degrees equals the cosine of -180
degrees equals -1. Odd functions change sign, for example the sine of 90 degrees equals 1, but the sine of
-90 degrees equals -1. The same is true of the wave functions describing particles. If the sign is unchanged by
a parity transform, then the particle is said to have even parity, or a parity of +1, for example the parity of the
proton is +1. If the wave function changes sign then the particle has odd parity (a parity of -1), for example, the
photon and the pion (pi meson). [There are thus two eigenvalues of the parity operator: +1 and -1.] Parity is
thus an intrinsic property of elementary particles. Anti-particles have opposite parity (opposite in sign) to their
corresponding particles. Applying parity transformations twice in succession returns the original system, i.e.
leaves the system unchanged.
Charge Conjugation
Charge conjugation (C) is a type of theoretic transformation in which all particles are replaced by their anti-
particles (and anti-particles by their particles) whilst leaving other properties unchanged. This changes the
sign of the particle’s electric charge, parity and magnetic moment, but leaves properties like momentum and
position unchanged.
Again for the strong and electromagnetic forces, charge conjugation is conserved:

However, this conservation (called C-invariance) is violated again by the weak force. Similar to parity, the
eigenvalues for the charge conjugation operator are +1 and -1. Applying the operator again returns the
system back to its original state.
Time Reversal
Time reversal involves replacing all the time coordinates, t, by –t. In other words, we reverse the process as if
it was running backwards in time. Many processes work just the same in reverse, e.g. think of a gamma-ray
photon turning into a positron-electron pair. Now reverse this and we have the annihilation of the pair to
produce a photon, something which is physically feasible and the forward rate of reaction should equal the
reverse rate. The electromagnetic force, as described by QED, and the strong force, as described by QCD,
are both time-reversible – reactions proceed just as quickly in reverse as they do forwards (this is a familiar
concept in chemistry in which many reactions are reversible).
However, not all processes are symmetrical when time-forward is compared to time-reversed. reactions
involve the weak force violate time-reversal symmetry, these reactions do not occur with equal rate in both
directions. The weak force will be covered in a future article, however, it involves the helicity of particles and
helices do not necessarily have the same properties when reversed. Try opening a bottle of wine by turning
the corkscrew in the wrong direction, or undoing a screw or opening a tap by turning in the wrong direction!
[The fact that a helix rotating about its long axis is not time-reversible explains how bacterial flagella work in
highly viscous fluids.] Time reversal is different to the spatial symmetries, however, in that it does not lead to a
conserved (or approximately conserved) observable quantity, since the time-reversal operator does not have
the necessary properties to yield observable eigenvalues.
Gauge Invariance
Electric and magnetic fields can be expressed mathematically as functions of a scalar field and a vector field.
(A scalar field is one in which a number is associated with each point in space, e.g. temperature in a
temperature field. A vector field is one in which a vector, that is a number and a direction, is associated with
each point in space, e.g. the gravitational field which points towards the centre of large objects such as the
Earth and grows in strength as you approach the object.)
Think of how the electric field surrounding an electric charge has a value of field strength at each point in
space, but also how direction is important – a moving electric charge, for example, generates a magnetic field,
and moving electric charges follow helical paths in magnetic fields (see particle paths).
Since two fields are involved (scalar and vector) it is possible to change (transform) these two fields by adding
specific mathematical terms to them without changing the overall electric and magnetic fields. Only the electric
and magnetic fields are observable, not the component scalar and vector fields (which are useful
mathematical devices).
In a gauge transformation, the underlying mathematical components, such as the scalar and vector fields
for the electromagnetic force, are changed without changing the observable properties of the system. Such a
transformation achieves no observable change in the physics, rather like our translations of the coordinates
in space, and is similarly due to a symmetry called gauge invariance. These transformations are global -
they apply to all points in space and time. (We will consider local symmetries later). Think of voltage, which is
the difference in electric potential across two points in space, such as across the terminals of a power cell or
battery. This voltage or potential difference (p.d.) drives electric current (the flow of electric charge) around a
circuit. It is the potential difference that matters, not the actual potential. A squirrel can walk across a high-
voltage power line without frying, because there is no p.d. across its feet: the potential is the same
everywhere along the cable, but if it had one foot on the line and another on the ground then it has problems!
We could globally change the electric potential everywhere in space and time and nobody would notice! This
is a consequence of a global symmetry (which leads to the conservation of electric charge). It is a bit like
changing the gauge of a rail network (the width between the rails) - as long as all lines use the same gauge it
is of no consequence to th running of the trains.
Changing the electric and magnetic fields by a gauge transformation does not change the wave equation
(Schrodinger’s or Dirac’s) if a compensating change can be made to the wave function which also does not
change the physics. Remember, that if a particle is in an electric or magnetic field that this will enter the
Hamiltonian in the potential energy term and so change the wave equation.
A free particle is one that is not moving in an observable potential difference, that is the force-fields and
potentials it are moving to are all constant and this constant value can be taken to be zero. (It is like an
electric battery – it is the potential difference or voltage across the terminals that drives the electric current
around a circuit, the actual value of the potential has no physical meaning and can be taken as zero – no
current flows if only one terminal is in contact with the circuit.) The time-dependent wave-function for such a
free particle can be written as the time-independent wave-function multiplied by an exponential phase factor
that describes the wave-like oscillations in time.
eigenvalues for the charge conjugation operator are +1 and -1. Applying the operator again returns the
system back to its original state.
Time Reversal
Time reversal involves replacing all the time coordinates, t, by –t. In other words, we reverse the process as if
it was running backwards in time. Many processes work just the same in reverse, e.g. think of a gamma-ray
photon turning into a positron-electron pair. Now reverse this and we have the annihilation of the pair to
produce a photon, something which is physically feasible and the forward rate of reaction should equal the
reverse rate. The electromagnetic force, as described by QED, and the strong force, as described by QCD,
are both time-reversible – reactions proceed just as quickly in reverse as they do forwards (this is a familiar
concept in chemistry in which many reactions are reversible).
However, not all processes are symmetrical when time-forward is compared to time-reversed. reactions
involve the weak force violate time-reversal symmetry, these reactions do not occur with equal rate in both
directions. The weak force will be covered in a future article, however, it involves the helicity of particles and
helices do not necessarily have the same properties when reversed. Try opening a bottle of wine by turning
the corkscrew in the wrong direction, or undoing a screw or opening a tap by turning in the wrong direction!
[The fact that a helix rotating about its long axis is not time-reversible explains how bacterial flagella work in
highly viscous fluids.] Time reversal is different to the spatial symmetries, however, in that it does not lead to a
conserved (or approximately conserved) observable quantity, since the time-reversal operator does not have
the necessary properties to yield observable eigenvalues.
Gauge Invariance
Electric and magnetic fields can be expressed mathematically as functions of a scalar field and a vector field.
(A scalar field is one in which a number is associated with each point in space, e.g. temperature in a
temperature field. A vector field is one in which a vector, that is a number and a direction, is associated with
each point in space, e.g. the gravitational field which points towards the centre of large objects such as the
Earth and grows in strength as you approach the object.)
Think of how the electric field surrounding an electric charge has a value of field strength at each point in
space, but also how direction is important – a moving electric charge, for example, generates a magnetic field,
and moving electric charges follow helical paths in magnetic fields (see particle paths).
Since two fields are involved (scalar and vector) it is possible to change (transform) these two fields by adding
specific mathematical terms to them without changing the overall electric and magnetic fields. Only the electric
and magnetic fields are observable, not the component scalar and vector fields (which are useful
mathematical devices).
In a gauge transformation, the underlying mathematical components, such as the scalar and vector fields
for the electromagnetic force, are changed without changing the observable properties of the system. Such a
transformation achieves no observable change in the physics, rather like our translations of the coordinates
in space, and is similarly due to a symmetry called gauge invariance. These transformations are global -
they apply to all points in space and time. (We will consider local symmetries later). Think of voltage, which is
the difference in electric potential across two points in space, such as across the terminals of a power cell or
battery. This voltage or potential difference (p.d.) drives electric current (the flow of electric charge) around a
circuit. It is the potential difference that matters, not the actual potential. A squirrel can walk across a high-
voltage power line without frying, because there is no p.d. across its feet: the potential is the same
everywhere along the cable, but if it had one foot on the line and another on the ground then it has problems!
We could globally change the electric potential everywhere in space and time and nobody would notice! This
is a consequence of a global symmetry (which leads to the conservation of electric charge). It is a bit like
changing the gauge of a rail network (the width between the rails) - as long as all lines use the same gauge it
is of no consequence to th running of the trains.
Changing the electric and magnetic fields by a gauge transformation does not change the wave equation
(Schrodinger’s or Dirac’s) if a compensating change can be made to the wave function which also does not
change the physics. Remember, that if a particle is in an electric or magnetic field that this will enter the
Hamiltonian in the potential energy term and so change the wave equation.
A free particle is one that is not moving in an observable potential difference, that is the force-fields and
potentials it are moving to are all constant and this constant value can be taken to be zero. (It is like an
electric battery – it is the potential difference or voltage across the terminals that drives the electric current
around a circuit, the actual value of the potential has no physical meaning and can be taken as zero – no
current flows if only one terminal is in contact with the circuit.) The time-dependent wave-function for such a
free particle can be written as the time-independent wave-function multiplied by an exponential phase factor
that describes the wave-like oscillations in time.

The wave-function itself does not correspond directly to the observable properties of the particle, instead it is
the square of the wave function, which gives us the probability that the particle will be found in a particular
region of space (and for example gives us the hydrogen atomic orbitals). When we square the wave-function,
the exponential terms vanish, due to the presences of i, the square-root of -1, which makes the wave-function
complex. See the box below for information on squaring complex numbers and wave functions.
the square of the wave function, which gives us the probability that the particle will be found in a particular
region of space (and for example gives us the hydrogen atomic orbitals). When we square the wave-function,
the exponential terms vanish, due to the presences of i, the square-root of -1, which makes the wave-function
complex. See the box below for information on squaring complex numbers and wave functions.

Squaring the wave-function removes the time-dependent exponential factors (called phase-factors). This
means that many different phase-factors may correspond to the same physical state. This allows us to
compensate for a change in gauge (a gauge transformation) by changing the phase-factor in some way so
as to keep the wave equation (Schrodinger or Dirac wave equation) invariant - meaning that the gauge
transformation has no effect on it and no effect on the physics.
Gauge Principle
The gauge principle states that if we reverse the above process then we can learn a lot about the nature of
the forces governing the potential in the Hamiltonian. We can begin by transforming the wave-function and
then seeing what (minimum) change (gauge transformation) in the mathematical terms describing the
potential energy (part of the Hamiltonian) are necessary to keep the wave equation gauge invariant. For
example, if we transform the wave-function and then plug into the Dirac equation for an electron, then we
obtain the physics of quantum electrodynamics (QED) which explains the electromagnetic force! Similarly
for quantum chromodynamics (QCD) which describes the strong force.
Higgs Boson
The current or standard model of particle physics predicts the existence of a spin-0 boson (meaning that
its intrinsic angular momentum, or spin, is zero) called the Higgs boson. There are two main classes of
particles - fermions and bosons. They differ in the way they interact with one-another (the statistics
describing the behaviour of populations of these particles differ). The standard model describes four
fundamental forces that can act between particles, causing them to repel one-another or be attracted to
one-another, and each force is due to the exchange of force-carrying particles, all types of boson, called
gauge bosons, between the interacting particles:
1. The electromagnetic force, conveyed by photons, described by QED.
2. The strong force, conveyed by gluons, described by QCD.
3. The weak force, conveyed by charged W+ and W- bosons and neutral Z bosons.
4. Gravity, thought to be conveyed by gravitons, not yet fully described.
These gauge bosons are all spin-1 particles. However, gauge invariance predicts that if all the force-carriers
are spin-1 then they must be massless. Photons and gluons are certainly massless, but W+, W- and z
bosons are not, these are very heavy particles! This can be explained by the hypothesised existence of the
Higgs boson, which interacts with all the gauge bosons, but most strongly with those that have mass (the
weak force bosons) allowing them to acquire mass without violating gauge invatiance. In some ways the
Higgs boson would behave like a fifth fundamental interaction.
The Search for the Higgs Boson and the LHC
The Higgs boson has not yet been definitely discovered (as of 1/1/2012). The problem is that it is not easily
produced and is predicted to be quite rare even at very high energies. The European particle research
facility, CERN (external link: CERN) have been searching for it for years. In the past they used their large
electron-positron collider (LEP) to smash beams of electrons and positrons together at very high
energies. An example of such a collision is shown below:
means that many different phase-factors may correspond to the same physical state. This allows us to
compensate for a change in gauge (a gauge transformation) by changing the phase-factor in some way so
as to keep the wave equation (Schrodinger or Dirac wave equation) invariant - meaning that the gauge
transformation has no effect on it and no effect on the physics.
Gauge Principle
The gauge principle states that if we reverse the above process then we can learn a lot about the nature of
the forces governing the potential in the Hamiltonian. We can begin by transforming the wave-function and
then seeing what (minimum) change (gauge transformation) in the mathematical terms describing the
potential energy (part of the Hamiltonian) are necessary to keep the wave equation gauge invariant. For
example, if we transform the wave-function and then plug into the Dirac equation for an electron, then we
obtain the physics of quantum electrodynamics (QED) which explains the electromagnetic force! Similarly
for quantum chromodynamics (QCD) which describes the strong force.
Higgs Boson
The current or standard model of particle physics predicts the existence of a spin-0 boson (meaning that
its intrinsic angular momentum, or spin, is zero) called the Higgs boson. There are two main classes of
particles - fermions and bosons. They differ in the way they interact with one-another (the statistics
describing the behaviour of populations of these particles differ). The standard model describes four
fundamental forces that can act between particles, causing them to repel one-another or be attracted to
one-another, and each force is due to the exchange of force-carrying particles, all types of boson, called
gauge bosons, between the interacting particles:
1. The electromagnetic force, conveyed by photons, described by QED.
2. The strong force, conveyed by gluons, described by QCD.
3. The weak force, conveyed by charged W+ and W- bosons and neutral Z bosons.
4. Gravity, thought to be conveyed by gravitons, not yet fully described.
These gauge bosons are all spin-1 particles. However, gauge invariance predicts that if all the force-carriers
are spin-1 then they must be massless. Photons and gluons are certainly massless, but W+, W- and z
bosons are not, these are very heavy particles! This can be explained by the hypothesised existence of the
Higgs boson, which interacts with all the gauge bosons, but most strongly with those that have mass (the
weak force bosons) allowing them to acquire mass without violating gauge invatiance. In some ways the
Higgs boson would behave like a fifth fundamental interaction.
The Search for the Higgs Boson and the LHC
The Higgs boson has not yet been definitely discovered (as of 1/1/2012). The problem is that it is not easily
produced and is predicted to be quite rare even at very high energies. The European particle research
facility, CERN (external link: CERN) have been searching for it for years. In the past they used their large
electron-positron collider (LEP) to smash beams of electrons and positrons together at very high
energies. An example of such a collision is shown below:
This is a one-jet event with the Z-boson converting into a jet of hadrons.
Below is an example of the type of expected interaction between a Z-boson and a Higgs boson.
Below is an example of the type of expected interaction between a Z-boson and a Higgs boson.


Currently a number of experiments are underway at CERN, using the large hadron collider (LHC) (external
link: LHC) which is the largest and most powerful particle-accelerator on Earth (indeed it is the largest
machine constructed by Earthlings). This accelerator collides beams of hadrons, in this case either protons
or lead nuclei, at immense energies, recreating conditions very shortly after the Big Bang (but of course on a
much smaller scale!). One such experiment hopes to detect the Higgs boson! Time will tell.
Exchange Symmetry - The Consequences of Identity
A very important and interesting phenomenon manifests when one extends the study of the hydrogen atom
to a helium atom. Hydrogen atoms consist of a single electron moving in a Coulomb potential well or force
field and the motion of the electron can be described by Schrodinger's Wave equation (with an additional
relativistic correction and other small corrections added if such accuracy is required). In heavier atoms,
relativistic effects may become more important, but another profound difference becomes apparent.
Consider a helium atom, He, in which 2 electrons now orbit the nucleus. If the electrons were like billiard balls,
then we could label one as ball A and the other as ball B and track the balls so that we know at all times
which is which - the balls are distinguishable since they are two separate objects. In QM particles like
electrons can not follow definite trajectories (the Heisenberg Uncertainty Principle forbids it) since their
positions and momenta cannot be both precisely known - there are no trajectories in QM (assuming there are
no 'hidden variables'). Now we have an interesting effect indeed: the two electrons in a He atom are so close
together that their wavefunctions (or the uncertainties in their positions) overlap and it is impossible in
principle to distinguish one electron from the other. The electrons behave and indeed become a single entity
or state. They lose their identities!
What we require is:
link: LHC) which is the largest and most powerful particle-accelerator on Earth (indeed it is the largest
machine constructed by Earthlings). This accelerator collides beams of hadrons, in this case either protons
or lead nuclei, at immense energies, recreating conditions very shortly after the Big Bang (but of course on a
much smaller scale!). One such experiment hopes to detect the Higgs boson! Time will tell.
Exchange Symmetry - The Consequences of Identity
A very important and interesting phenomenon manifests when one extends the study of the hydrogen atom
to a helium atom. Hydrogen atoms consist of a single electron moving in a Coulomb potential well or force
field and the motion of the electron can be described by Schrodinger's Wave equation (with an additional
relativistic correction and other small corrections added if such accuracy is required). In heavier atoms,
relativistic effects may become more important, but another profound difference becomes apparent.
Consider a helium atom, He, in which 2 electrons now orbit the nucleus. If the electrons were like billiard balls,
then we could label one as ball A and the other as ball B and track the balls so that we know at all times
which is which - the balls are distinguishable since they are two separate objects. In QM particles like
electrons can not follow definite trajectories (the Heisenberg Uncertainty Principle forbids it) since their
positions and momenta cannot be both precisely known - there are no trajectories in QM (assuming there are
no 'hidden variables'). Now we have an interesting effect indeed: the two electrons in a He atom are so close
together that their wavefunctions (or the uncertainties in their positions) overlap and it is impossible in
principle to distinguish one electron from the other. The electrons behave and indeed become a single entity
or state. They lose their identities!
What we require is:

Note that the composite state of the two particles (e.g. electrons in He) is obtained by multiplying together their
individual wavefunctions. This expression tells us that the state is unchanged if we interchange the two
particles A and B. This is what must hold as the two electrons are indistinguishable.
However, if we consider a standard QM system such as infinite square well (in which particles are trapped in a
potential well of infinite depth, that is a force-field of infinite strength, which is square-shaped) then
interchanging the two particles does not result in an unchanged state. (An infinite square well is a
mathematical simplification to demonstrate the solution of wave equations in a relatively simple system,
however, it is an approximation that can be rarely applied in real-life; for our purposes the shape of the well
does not matter, we are simply illustrating a general point). This is illustrated diagrammatically below:
individual wavefunctions. This expression tells us that the state is unchanged if we interchange the two
particles A and B. This is what must hold as the two electrons are indistinguishable.
However, if we consider a standard QM system such as infinite square well (in which particles are trapped in a
potential well of infinite depth, that is a force-field of infinite strength, which is square-shaped) then
interchanging the two particles does not result in an unchanged state. (An infinite square well is a
mathematical simplification to demonstrate the solution of wave equations in a relatively simple system,
however, it is an approximation that can be rarely applied in real-life; for our purposes the shape of the well
does not matter, we are simply illustrating a general point). This is illustrated diagrammatically below:



These graphs show the wave-functions of the two particles, which are occupying the two lowest energy
states (the lowest has no nodes or points where it crosses the x-axis, the second lowest has one node,
the third lowest would have 2 nodes, etc, so that the frequency of the wave increases as its energy
increases). The only change we have made is to swap the positions (x1 and x2) of the two particles,
however, the two states are clearly different, so particle exchange symmetry has been violated.
The way around this is to actually describe the particle states using linear combinations of the two
wavefunctions, which is possible since we are dealing with (linear) waves and it is possible to add waves
together to get a new wave, both the original waves and the new composite waves will satisfy our wave
equation and so both are permissible. The simplest combination that works is:
states (the lowest has no nodes or points where it crosses the x-axis, the second lowest has one node,
the third lowest would have 2 nodes, etc, so that the frequency of the wave increases as its energy
increases). The only change we have made is to swap the positions (x1 and x2) of the two particles,
however, the two states are clearly different, so particle exchange symmetry has been violated.
The way around this is to actually describe the particle states using linear combinations of the two
wavefunctions, which is possible since we are dealing with (linear) waves and it is possible to add waves
together to get a new wave, both the original waves and the new composite waves will satisfy our wave
equation and so both are permissible. The simplest combination that works is:
One combination is symmetric, since it results in no change to the wave-function, whereas the
antisymmetric combination results in a change of sign of the wave-function (i.e. if it is positive then
exchanging particles makes it negative, if negative then exchange makes it positive). However, since
what we observe is the probability density, obtained by squaring the wave-function (taking the square
of the modulus) the sign of the wave-function makes no difference (consider -2 x -2 = 2 x 2 = 4). Thus,
both combinations are acceptable solutions. The 1/square-root of 2 multiplying factor ensures that
when we square the wave-functions the total probability adds to 1, as it must (the probability of finding
the particles somewhere has to be zero) and is called a normalisation factor.
Plotting the probability distributions for a pair of identical particles, at positions x1 and x2 in our infinite
square well, in the symmetric state gives the following, where red indicates higher probability, blue
lower probability of finding the particles:
antisymmetric combination results in a change of sign of the wave-function (i.e. if it is positive then
exchanging particles makes it negative, if negative then exchange makes it positive). However, since
what we observe is the probability density, obtained by squaring the wave-function (taking the square
of the modulus) the sign of the wave-function makes no difference (consider -2 x -2 = 2 x 2 = 4). Thus,
both combinations are acceptable solutions. The 1/square-root of 2 multiplying factor ensures that
when we square the wave-functions the total probability adds to 1, as it must (the probability of finding
the particles somewhere has to be zero) and is called a normalisation factor.
Plotting the probability distributions for a pair of identical particles, at positions x1 and x2 in our infinite
square well, in the symmetric state gives the following, where red indicates higher probability, blue
lower probability of finding the particles:



The dark-blue diagonal line shows us where the probability of finding either particle is zero. If both
particles occupy the same position in space, then x1 = x2 and the particles are somewhere on the
dashed diagonal-line, which passes through the red areas where the particles are very likely to be find
- that is the particles are more likely to be found close together. In other words, when two particles
are in a symmetric state, they tend to 'huddle together'.
The equivalent plot for two particles in the antisymmetric state gives the following:
particles occupy the same position in space, then x1 = x2 and the particles are somewhere on the
dashed diagonal-line, which passes through the red areas where the particles are very likely to be find
- that is the particles are more likely to be found close together. In other words, when two particles
are in a symmetric state, they tend to 'huddle together'.
The equivalent plot for two particles in the antisymmetric state gives the following:
This time when the particles are together, with x1 = x2, they lie along the dark-blue line which is
impossible, since this is a region of zero probability! In the antisymmetric state, the two particles
tend to avoid one-another.
A mysterious quantum-mechanical force has appeared, causing the particles to move closer together
if they are symmetric, or to repel if they are antisymmetric. This is the exchange force and results
purely from the fact that the two particles are indistinguishable.
Whether or not particles are symmetric or antisymmetric is a fundamental property of the particle
type. Electrons, neutrons and protons, and composite particles containing an odd-number of these
particles, e.g. a He-3 atom (two electrons, two protons and one neutron) are antisymmetric and these
are called fermions. Particles like photons and composite particles containing an even-number of
fermions, e.g. the He-4 atom (two electrons, two protons and two neutrons) are symmetric and are
called bosons.
The ability of bosons to huddle together can give rise to some very strange effects. When liquid He-4
is cooled to near absolute zero, the wave-functions of the atoms extend and overlap so that the
whole body of liquid behaves as a single quantum state. It becomes a superfluid, flowing with zero
friction, flowing uphill as easily as downhill. Furthermore, if a ladle of such liquid is withdrawn from a
vessel, then the liquid in the ladle flows up over the sides and back into the vessel, so that the whole
liquid remains in the same state as a single entity (or as near as possible)! (Check out videos of this
on Youtube).
Electrons in an atom can pair-up, and have to in order to fill an atomic orbital which can hold two
electrons, but to do so they must be in different quantum states. In other words they can not have
the same set of values for all their quantum numbers, at least one must differ, and this may be the
spin, with one particle in the spin-up state, the other spin-down. Bosons have zero or integer spin.
For particles with spin, like electrons, it is actually the total wave-function which must be
antisymmetric. So far we have only considered spatial wave-functions, but the whole electron state
is described by a spatial wave-function multiplied by a spin wave-function. One, and only one, of
these must be antisymmetric to make the total wave-function antisymmetric. Thus electrons can exist
in a symmetric spatial state if their spin states are antisymmetric, and they can be in an antisymmetric
spatial state if their spin state is symmetric:
impossible, since this is a region of zero probability! In the antisymmetric state, the two particles
tend to avoid one-another.
A mysterious quantum-mechanical force has appeared, causing the particles to move closer together
if they are symmetric, or to repel if they are antisymmetric. This is the exchange force and results
purely from the fact that the two particles are indistinguishable.
Whether or not particles are symmetric or antisymmetric is a fundamental property of the particle
type. Electrons, neutrons and protons, and composite particles containing an odd-number of these
particles, e.g. a He-3 atom (two electrons, two protons and one neutron) are antisymmetric and these
are called fermions. Particles like photons and composite particles containing an even-number of
fermions, e.g. the He-4 atom (two electrons, two protons and two neutrons) are symmetric and are
called bosons.
- Fermions, like electrons, are antisymmetric and tend to avoid one-another and must occupy
different quantum states. - Bosons, like photons, are symmetric and tend to huddle together and can occupy the same
quantum state.
The ability of bosons to huddle together can give rise to some very strange effects. When liquid He-4
is cooled to near absolute zero, the wave-functions of the atoms extend and overlap so that the
whole body of liquid behaves as a single quantum state. It becomes a superfluid, flowing with zero
friction, flowing uphill as easily as downhill. Furthermore, if a ladle of such liquid is withdrawn from a
vessel, then the liquid in the ladle flows up over the sides and back into the vessel, so that the whole
liquid remains in the same state as a single entity (or as near as possible)! (Check out videos of this
on Youtube).
Electrons in an atom can pair-up, and have to in order to fill an atomic orbital which can hold two
electrons, but to do so they must be in different quantum states. In other words they can not have
the same set of values for all their quantum numbers, at least one must differ, and this may be the
spin, with one particle in the spin-up state, the other spin-down. Bosons have zero or integer spin.
For particles with spin, like electrons, it is actually the total wave-function which must be
antisymmetric. So far we have only considered spatial wave-functions, but the whole electron state
is described by a spatial wave-function multiplied by a spin wave-function. One, and only one, of
these must be antisymmetric to make the total wave-function antisymmetric. Thus electrons can exist
in a symmetric spatial state if their spin states are antisymmetric, and they can be in an antisymmetric
spatial state if their spin state is symmetric:
If more than two particles are being considered then symmetric multiplets are possible.
Since the total wave-function must be antisymmetric, two electrons in the singlet state (spins
antiparallel) will tend to huddle together, since their spatial wave-function must be symmetric.
Two electrons in a triplet state (spins parallel) will tend to avoid one-another, since their
spatial wave-function must be antisymmetric.
Another force exists between the two electrons in a He atom: Coulomb (electric) repulsion, both
electrons are negatively charged and negative charges repel one-another. This means that when
two electrons are in a symmetric spatial state, that is a singlet spin state and huddled together, the
energy of repulsion is higher and the energy of such states is slightly higher than for triplet states.
This is why electrons will tend to spread out within a shell by initially occupying orbitals singly with
their spins parallel, before pairing up (see atomic structure). This difference in energies is called the
exchange energy.
We also now have the basis for the Pauli exclusion principle: no two electrons in the same atom
can have all quantum numbers the same.
Group Theory and Isospin
Symmetries are often described by mathematical constructs called groups. To understand what a
mathematical group is, first look at the Latin squares below:
Since the total wave-function must be antisymmetric, two electrons in the singlet state (spins
antiparallel) will tend to huddle together, since their spatial wave-function must be symmetric.
Two electrons in a triplet state (spins parallel) will tend to avoid one-another, since their
spatial wave-function must be antisymmetric.
Another force exists between the two electrons in a He atom: Coulomb (electric) repulsion, both
electrons are negatively charged and negative charges repel one-another. This means that when
two electrons are in a symmetric spatial state, that is a singlet spin state and huddled together, the
energy of repulsion is higher and the energy of such states is slightly higher than for triplet states.
This is why electrons will tend to spread out within a shell by initially occupying orbitals singly with
their spins parallel, before pairing up (see atomic structure). This difference in energies is called the
exchange energy.
We also now have the basis for the Pauli exclusion principle: no two electrons in the same atom
can have all quantum numbers the same.
Group Theory and Isospin
Symmetries are often described by mathematical constructs called groups. To understand what a
mathematical group is, first look at the Latin squares below:

The situation becomes more complex with quarks, which are also fermions with spin. Quarks have a
third component to their total wave-function: the colour wave-function (see QED) and either one or
all three of these components must be negative to make their state antisymmetric, as it must be.
[The way to remember this is to think of each antisymmetric component wave-function as -1 and
each symmetric one as +1, then for a quark: -1 x +1 x +1 = -1 which is antisymmetric, as does -1 x -1
x -1 = -1).
It turns out that for two electrons there are three symmetric states, called a triplet, corresponding to
both electrons being spin-up, both being spin-down and a combination of one spin-up and the other
spin-down, such that the total spin is S = 1 in both cases (both spins aligned parallel). There is one
antisymmetric spin-state, called a singlet, which is also a combination, such that S = 0 (spins
aligned antiparallel). They are as follows:
third component to their total wave-function: the colour wave-function (see QED) and either one or
all three of these components must be negative to make their state antisymmetric, as it must be.
[The way to remember this is to think of each antisymmetric component wave-function as -1 and
each symmetric one as +1, then for a quark: -1 x +1 x +1 = -1 which is antisymmetric, as does -1 x -1
x -1 = -1).
It turns out that for two electrons there are three symmetric states, called a triplet, corresponding to
both electrons being spin-up, both being spin-down and a combination of one spin-up and the other
spin-down, such that the total spin is S = 1 in both cases (both spins aligned parallel). There is one
antisymmetric spin-state, called a singlet, which is also a combination, such that S = 0 (spins
aligned antiparallel). They are as follows:
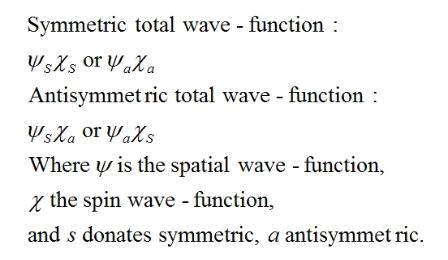
Coming soon...
Grand Unification
Supersymmetry
Grand Unification
Supersymmetry

Each element in the square appears once, and once only, in each row, and the order of elements is
change din each row to give all possible orders. A Latin square gives the rules for multiplying its
elements together, in this case the elements e, p and q. So, to obtain p x q or pq for the left-hand
square, look up the first element, p, across the top, then move down the column to the second
element, q, and you obtain the answer: pq = e. Check that ep = p and pe = p. For the right-hand
square you will see that ep = q and pe = e.
Notice that for the left-hand square, element e is an identity element, since ex = xe = x for any
other element x. For the left-hand square (but not the right-hand one) element e is an identity
element, since: ep = pe = p, eq = qe = q, and ee = e.
A group is a Latin square that satisfies two conditions: 1) it has an identity element, 2) it obeys the
associative law of algebra when multiplying together 3 or more elements, that is: x(yz) = (xy)z for
elements, x, y and z. Note that the order of multiplication, however, can matter, for example, in the
right-hand square, pq = q, but qp = p (though this square is not a group as it has no identity
element).
As an example of a symmetry group, consider the rhombus below:
change din each row to give all possible orders. A Latin square gives the rules for multiplying its
elements together, in this case the elements e, p and q. So, to obtain p x q or pq for the left-hand
square, look up the first element, p, across the top, then move down the column to the second
element, q, and you obtain the answer: pq = e. Check that ep = p and pe = p. For the right-hand
square you will see that ep = q and pe = e.
Notice that for the left-hand square, element e is an identity element, since ex = xe = x for any
other element x. For the left-hand square (but not the right-hand one) element e is an identity
element, since: ep = pe = p, eq = qe = q, and ee = e.
A group is a Latin square that satisfies two conditions: 1) it has an identity element, 2) it obeys the
associative law of algebra when multiplying together 3 or more elements, that is: x(yz) = (xy)z for
elements, x, y and z. Note that the order of multiplication, however, can matter, for example, in the
right-hand square, pq = q, but qp = p (though this square is not a group as it has no identity
element).
- In an Abelian group, the order of multiplication does not matter (like multiplying together
ordinary numbers (for which 1 is the identity element). - In a non-Abelian group the order of multiplication does matter, we shall see an example later.
As an example of a symmetry group, consider the rhombus below:

The rhombus has the symmetries listed, that is each of those operations leaves the rhombus unchanged, for
example a rotation of 180 degrees about its centre (a rotation of 90 degrees would leave the narrower blue
angles vertical and so change the appearance of the rhombus). (One has to be careful with rotations and
reflections, since often the order in which a series of two or more rotations is carried out can make a
difference. For example, consider the point x=1, y=1 or as an (x,y) pair: (1,1), now reflect this in the x-axis to
give (1,-1) and then rotate 90 degrees anticlockwise to give (1,1) again; reverse the operation: rotate 90
degrees anticlockwise, to give (-1,1), reflect in the x-axis and we have (-1,-1), so our two answers are
different! We say that these two operations did not commute.)
These 4 symmetry operations give the complete list of single reflections and rotations that leave the rhombus
unchanged. These geometrical operations form a group of transformation operators (a geometrical
transformation is any operation that changes position, shape or orientation) as shown below:
example a rotation of 180 degrees about its centre (a rotation of 90 degrees would leave the narrower blue
angles vertical and so change the appearance of the rhombus). (One has to be careful with rotations and
reflections, since often the order in which a series of two or more rotations is carried out can make a
difference. For example, consider the point x=1, y=1 or as an (x,y) pair: (1,1), now reflect this in the x-axis to
give (1,-1) and then rotate 90 degrees anticlockwise to give (1,1) again; reverse the operation: rotate 90
degrees anticlockwise, to give (-1,1), reflect in the x-axis and we have (-1,-1), so our two answers are
different! We say that these two operations did not commute.)
These 4 symmetry operations give the complete list of single reflections and rotations that leave the rhombus
unchanged. These geometrical operations form a group of transformation operators (a geometrical
transformation is any operation that changes position, shape or orientation) as shown below:

This group is an example of a general group called the Klein group, in which multiplying each element by
itself (the top-left to bottom-right diagonal) gives the identity element. Regular polygons, such as the
square, regular pentagon, hexagon, etc. form a special series of groups called dihedral groups. Other
geometric groups are important in chemistry, describing the symmetries of molecules and crystals, such
as the tetrahedral group, with 24 operations, describing the symmetry of tetrahedral molecules like
methane. The symmetry of an icosahedral virus is described by the icosahedral group which has 120
operations!
In particle/quantum physics, we have already seen that symmetries are crucial in describing and
discerning conservation laws and other physical laws. It is not surprising, therefore, that this field makes
use of symmetry groups. In this case the symmetries may not be groups of geometric operators operating
in real space, like our rotations and reflections of the rhombus, but they may be operating in an abstract
virtual space which models some aspect of the physics. As an example, consider isospin. This is a
mathematical property but it relates to real physical properties, in particular if electric charge is neglected,
then the proton and neutron behave almost like identical particles. This is called isospin symmetry,
exchanging protons and neutrons in a system leaves the physics essentially unchanged if one ignores the
electric charge. It is almost as if the proton and neutron were different states of the same particle. This
symmetry is only approximate, since the proton and neutron have slightly different masses (but only
slightly). This phenomenon is due to the similarities between the up and down quarks that make up the
proton and neutron. (A proton consists of 2 up (u) and one down (d) quark, whilst a neutron consists of
one u and 2 d quarks.) In particular, the u and d quarks have the same isospin (I = 1/2). Symmetry still
holds approximately if one includes the strange (s) quark, though this quark is quite heavy and so the
mass difference becomes more noticeable and this 3 quark symmetry is more approximate than u, d
quark isospin symmetry. Since the s quark has a different isospin (I = 0) this symmetry of 3 quarks is
called flavour symmetry, since u, d and s are all different flavours of quarks. Particles that are related
by isospin symmetry form an isospin multiplet.
In quantum mechanics, which is a mathematical model of physical systems, the state of the system in
consideration, that is its physical observable properties, is represented by a vector. Mathematical
operators multiply these vectors to produce a new vector, representing a new state. This operator could
be an angular momentum or energy (Hamiltonian) operator, representing some measurement of angular
momentum or energy (measurements change systems by interacting with them!) or some other physical
interaction. Sometimes, however, operators are abstract, such as the operators which replace all d quarks
by u or s quarks, for example (though as we shall see quarks can change flavour). This then allows us to
see what effect such a change has on the predicted behaviour of the system. (Like all scientific models,
quantum mechanics is useful because it makes accurate predictions). This is illustrated below:
itself (the top-left to bottom-right diagonal) gives the identity element. Regular polygons, such as the
square, regular pentagon, hexagon, etc. form a special series of groups called dihedral groups. Other
geometric groups are important in chemistry, describing the symmetries of molecules and crystals, such
as the tetrahedral group, with 24 operations, describing the symmetry of tetrahedral molecules like
methane. The symmetry of an icosahedral virus is described by the icosahedral group which has 120
operations!
In particle/quantum physics, we have already seen that symmetries are crucial in describing and
discerning conservation laws and other physical laws. It is not surprising, therefore, that this field makes
use of symmetry groups. In this case the symmetries may not be groups of geometric operators operating
in real space, like our rotations and reflections of the rhombus, but they may be operating in an abstract
virtual space which models some aspect of the physics. As an example, consider isospin. This is a
mathematical property but it relates to real physical properties, in particular if electric charge is neglected,
then the proton and neutron behave almost like identical particles. This is called isospin symmetry,
exchanging protons and neutrons in a system leaves the physics essentially unchanged if one ignores the
electric charge. It is almost as if the proton and neutron were different states of the same particle. This
symmetry is only approximate, since the proton and neutron have slightly different masses (but only
slightly). This phenomenon is due to the similarities between the up and down quarks that make up the
proton and neutron. (A proton consists of 2 up (u) and one down (d) quark, whilst a neutron consists of
one u and 2 d quarks.) In particular, the u and d quarks have the same isospin (I = 1/2). Symmetry still
holds approximately if one includes the strange (s) quark, though this quark is quite heavy and so the
mass difference becomes more noticeable and this 3 quark symmetry is more approximate than u, d
quark isospin symmetry. Since the s quark has a different isospin (I = 0) this symmetry of 3 quarks is
called flavour symmetry, since u, d and s are all different flavours of quarks. Particles that are related
by isospin symmetry form an isospin multiplet.
In quantum mechanics, which is a mathematical model of physical systems, the state of the system in
consideration, that is its physical observable properties, is represented by a vector. Mathematical
operators multiply these vectors to produce a new vector, representing a new state. This operator could
be an angular momentum or energy (Hamiltonian) operator, representing some measurement of angular
momentum or energy (measurements change systems by interacting with them!) or some other physical
interaction. Sometimes, however, operators are abstract, such as the operators which replace all d quarks
by u or s quarks, for example (though as we shall see quarks can change flavour). This then allows us to
see what effect such a change has on the predicted behaviour of the system. (Like all scientific models,
quantum mechanics is useful because it makes accurate predictions). This is illustrated below:
In this case, there are 8 matrices that can interchange the u, d and s quarks for one-another. This set of
matrix operators is a subset of the unitary group for 3 by 3 matrices (a unitary matrix has a special
mathematical property, which we wont worry about here (its a matrix whose Hermitian conjugate is equal
to the matrix's inverse, so it has a kind of symmetry itself). Of this unitary group, U(3), only 8 matrices are
physically relevant and they form the so-called special unitary group of 3 by 3 matrices, SU(3). If only u
and d quarks are considered, then we use the SU(2) group. These matrix operators transform
coordinates in isospin space, converting one isospin state into another (e.g. a u quark into a d quark). A
similar technique is used in geometry: we can represent geometric points by vectors and then rotations
and reflections of these points can be represented by matrices (transformation matrices) and
multiplying a vector by such a matrix may change its coordinates, producing a new vector. In the case of
isospin we have a virtual space rather than physical space, but it still relates to real physical properties.
Global and local symmetries
Having taken away electric charge, what is left that counts for isospin and flavour symmetries? The
strong force is independent of the electric charge, and it is the strong force that mainly binds quarks
together inside a proton or neutron, so isospin and flavour in genera are consequences of the strong
force. Global symmetries, like those of the SU(2) and SU(3) flavour groups, apply to all points in space
and time (at least in the system under consideration) and give rise to conservation laws. In the case of
SU(2) and SU(3) flavour symmetry groups this is the conservation of flavour. If the law was exact then
quarks could never change flavour, however, as d, u and s quarks differ in mass the law is not exact and
the electroweak force (or electromagnetism and the weak force combined) can break this
symmetry/conservation, e.g. by allowing a u quark to decay into a d quark (and a virtual W+ particle) with
a consequent net change in flavour.
We have seen that changing the scalar electric potential everywhere causes no observable change, it is
a global symmetry and ensures global electric charge conservation, in other words the total electric
charge in the Universe is constant. Similarly, in isospin invariance, globally exchanging all neutrons and
protrons (or equivalently all d and u quarks) would also have no observable effect and is another global
symmetry.
In addition to SU flavour symmetry groups, often donated SU(2)f and SU(3)f, there is an SU group
representing colour symmetry, in which colour charge is conserved. Quarks can carry three types of
colour charge, usually designated red(r), green(g) and blue(b). Colour charge is to the strong force what
electric charge is to the electromagnetic force (except in electromagnetic interactions there are only two
charge types: + and - ). Colour charges are sources of the strong interaction, as electric charges are
sources of the electromagnetic interaction. With three colour charges, the appropriate group of operators
governing colour charge is the SU(3)c group. Again colour charge conservation is only approximate.
The idea that electric charge and colour charge are conserved in the Universe as a whole is fine, but
unsatisfactory. Experiment shows that electric charge is also conserved locally, in a given experiment on
a small part of the Universe, perhaps a handful of particles. Local symmetries, or local gauge
symmetries, conserve conserved quantities locally by introducing compensating forces. Indeed, these
local gauge symmetries are the basis of the fundamental forces! When we talk of gauge symmetries
and gauge theories, we are usually referring to these local gauge symmetries, which ensure local
conservation and give rise to the forces of Nature! Understand these gauge symmetries and you
understood the fundamental forces that are carried by gauge bosons. For example, to conserve electric
charge in a single particle reaction, it is necessary to introduce another force, which turns out to be
magnetism! In this way electric and magnetic forces are combined into the single electromagnetic force!
(Note: special relativity also combines electricity and magnetism by a relativity symmetry).
Deriving a local invariance or symmetry from a global one involves: 1) inserting a 'charge' or coupling
constant to the equations; 2) making the transformation space and time-dependent. If the resulting
symmetry group is non-Abelian, then the carrier of the force, the gauge boson, is also a source of the
force or field.
The Electroweak Force and Grand Unification
Under construction...
matrix operators is a subset of the unitary group for 3 by 3 matrices (a unitary matrix has a special
mathematical property, which we wont worry about here (its a matrix whose Hermitian conjugate is equal
to the matrix's inverse, so it has a kind of symmetry itself). Of this unitary group, U(3), only 8 matrices are
physically relevant and they form the so-called special unitary group of 3 by 3 matrices, SU(3). If only u
and d quarks are considered, then we use the SU(2) group. These matrix operators transform
coordinates in isospin space, converting one isospin state into another (e.g. a u quark into a d quark). A
similar technique is used in geometry: we can represent geometric points by vectors and then rotations
and reflections of these points can be represented by matrices (transformation matrices) and
multiplying a vector by such a matrix may change its coordinates, producing a new vector. In the case of
isospin we have a virtual space rather than physical space, but it still relates to real physical properties.
- SU(2) is the group of operations related to u, d quark isospin symmetry;
- SU(3) is the group relating to u, d and s quark flavour symmetry.
- SU(2) is also a flavour symmetry, as d and u are flavours of quark and is sometimes called isospin
flavour symmetry. - These groups are non-Abelian and refer to global symmetries (symmetries that apply across space
and time.
Global and local symmetries
Having taken away electric charge, what is left that counts for isospin and flavour symmetries? The
strong force is independent of the electric charge, and it is the strong force that mainly binds quarks
together inside a proton or neutron, so isospin and flavour in genera are consequences of the strong
force. Global symmetries, like those of the SU(2) and SU(3) flavour groups, apply to all points in space
and time (at least in the system under consideration) and give rise to conservation laws. In the case of
SU(2) and SU(3) flavour symmetry groups this is the conservation of flavour. If the law was exact then
quarks could never change flavour, however, as d, u and s quarks differ in mass the law is not exact and
the electroweak force (or electromagnetism and the weak force combined) can break this
symmetry/conservation, e.g. by allowing a u quark to decay into a d quark (and a virtual W+ particle) with
a consequent net change in flavour.
We have seen that changing the scalar electric potential everywhere causes no observable change, it is
a global symmetry and ensures global electric charge conservation, in other words the total electric
charge in the Universe is constant. Similarly, in isospin invariance, globally exchanging all neutrons and
protrons (or equivalently all d and u quarks) would also have no observable effect and is another global
symmetry.
In addition to SU flavour symmetry groups, often donated SU(2)f and SU(3)f, there is an SU group
representing colour symmetry, in which colour charge is conserved. Quarks can carry three types of
colour charge, usually designated red(r), green(g) and blue(b). Colour charge is to the strong force what
electric charge is to the electromagnetic force (except in electromagnetic interactions there are only two
charge types: + and - ). Colour charges are sources of the strong interaction, as electric charges are
sources of the electromagnetic interaction. With three colour charges, the appropriate group of operators
governing colour charge is the SU(3)c group. Again colour charge conservation is only approximate.
The idea that electric charge and colour charge are conserved in the Universe as a whole is fine, but
unsatisfactory. Experiment shows that electric charge is also conserved locally, in a given experiment on
a small part of the Universe, perhaps a handful of particles. Local symmetries, or local gauge
symmetries, conserve conserved quantities locally by introducing compensating forces. Indeed, these
local gauge symmetries are the basis of the fundamental forces! When we talk of gauge symmetries
and gauge theories, we are usually referring to these local gauge symmetries, which ensure local
conservation and give rise to the forces of Nature! Understand these gauge symmetries and you
understood the fundamental forces that are carried by gauge bosons. For example, to conserve electric
charge in a single particle reaction, it is necessary to introduce another force, which turns out to be
magnetism! In this way electric and magnetic forces are combined into the single electromagnetic force!
(Note: special relativity also combines electricity and magnetism by a relativity symmetry).
Deriving a local invariance or symmetry from a global one involves: 1) inserting a 'charge' or coupling
constant to the equations; 2) making the transformation space and time-dependent. If the resulting
symmetry group is non-Abelian, then the carrier of the force, the gauge boson, is also a source of the
force or field.
- Quantum electrodynamics (QED) is the quantum theory of the electromagnetic field;
- It's gauge symmetry is described by the unitary matrix, U(1), which is Abelian;
- The electromagnetic force is carried by (virtual) photons (the gauge bosons);
- Photons are not sources of the elctromagnetic field - they have no electric charge.
- Quantum chromodynamics (QCD) is the quantum theory of the strong force or field;
- It's gauge symmetry is described by the SU(3) matrix for colour, which is non-Abelian;
- The strong force is carried by (virtual) gluons (the gauge bosons);
- Gluons are sources of the strong field - they carry colour charge.
The Electroweak Force and Grand Unification
Under construction...

Comment on this article!


